16.5 FOURIER TRANSFORMS FOR STEADY STATE ANALYSIS

---

For the previous mass/damper/spring problem we may now consider what happens if the force oscillates, instead of remaining constant after time=0sec.

To find the (steady state) response of the system for a particular frequency of excitation, we may use a Fourier transform. It involves replacing the Laplace `s' as shown below.

After the equation is in this form, the next step is to get it in terms of the real and imaginary components. To do this the complex components are eliminated from the denominator by multiplying with the complex conjugate.

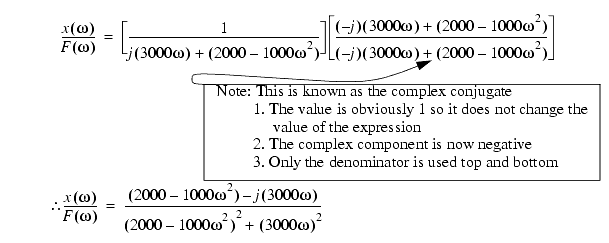
At this point the expression can be converted to a gain, and phase shift, by converting the real(x)/complex(y) components to polar coordinates (r,q).

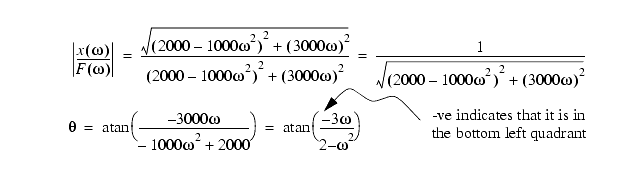
These can then be calculated, and plotted.

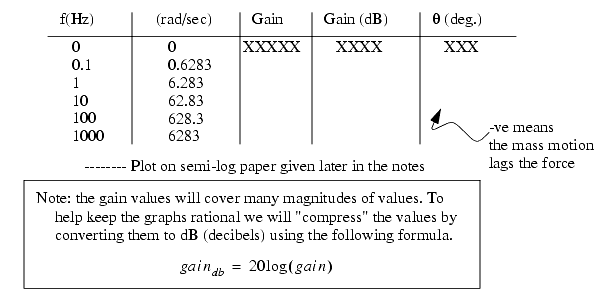
What does this mean? We can use the graph to find the gain and phase shift at various frequencies. In this example gain is defined as x/F. Therefore F is the input to the system, and x is the resulting output. The gain means that for each unit of F in, there will be gain*F=x out. The input and output are sinusoidal. There is a difference in phase between the input and output wave of q (the phase angle).

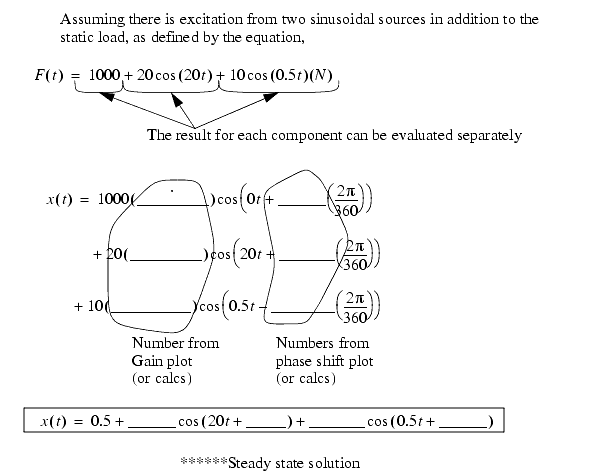
******** continue above with an example

Steady state solutions are valid after the system has been operating under the set condition for some time, and the initial transient effects have dissipated.