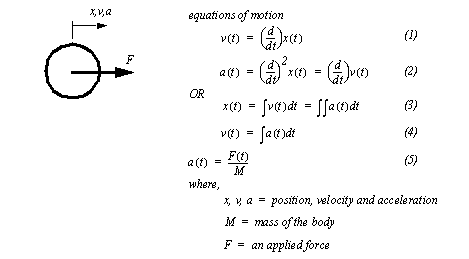
If the velocity and acceleration of a body are both zero then the body will be static. If the applied forces are balanced, and cancel each other out, the body will not accelerate. If the forces are unbalanced then the body will accelerate. If all of the forces act through the center of mass then the body will only translate. Forces that do not act through the center of mass will also cause rotation to occur. This chapter will focus only on translational systems.
The equations of motion for translating bodies are shown in Figure 2.1 Velocity and acceleration of a translating mass. These state simply that velocity is the first derivative of position, and acceleration is the first derivative of velocity with respect to time. Conversely the acceleration can be integrated to find velocity, and the velocity can be integrated to find position. Therefore, if we know the acceleration of a body, we can determine the velocity and position. Finally, when a force is applied to a mass, an acceleration can be found by dividing the net force by the mass.
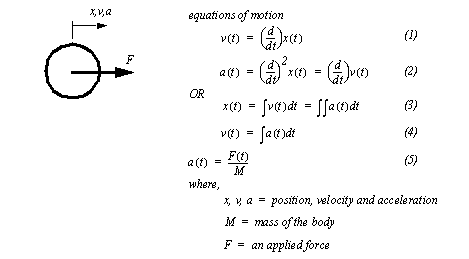
Figure 2.1 Velocity and acceleration of a translating mass
An example application of these fundamental laws is shown in Figure 2.2 Example: Calculation for a translating mass, with initial conditions. The initial conditions of the system are supplied (and are normally required to solve this type of problem). These are then used to find the state of the system after a period of time. The solution begins by integrating the acceleration, and using the initial velocity value for the integration constant. So at t=0 the velocity will be equal to the initial velocity. This is then integrated once more to provide the position of the object. As before, the initial position is used for the integration constant. This equation is then used to calculate the position after a period of time. Notice that the units are used throughout the calculations, this is a good practice for any engineer.
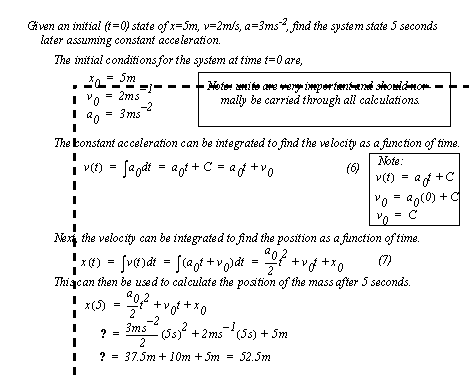
Figure 2.2 Example: Calculation for a translating mass, with initial conditions