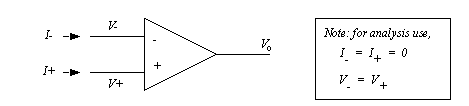
The ideal model of an op-amp is shown in Figure 7.27 An ideal op-amp. On the left hand side are the inverting and non-inverting inputs. Both of these inputs are assumed to have infinite impedance, and so no current will flow. Op-amp application circuits are designed so that the inverting and non-inverting inputs are driven to the same voltage level. The output of the op-amp is shown on the right. In circuits op-amps are used with feedback to perform standard operations such as those listed below.
• adders, subtractors, multipliers, and dividers - simple analog math operations
• amplifiers - increase the amplitude of a signal
• impedance isolators - hide the resistance of a circuit while passing a voltage

A simple op-amp example is given in Figure 7.28 A simple inverting operational amplifier configuration. As expected both of the op-amp input voltages are the same. This is a function of the circuit design. (Note: most op-amp circuits are designed to force both inputs to have the same voltage, so it is normally reasonable to assume they are the same.) The non-inverting input is connected directly to ground, so it will force both of the inputs to 0V. When the currents are summed at the inverting input, an equation including the input and output voltages is obtained. The final equation shows the system is a simple multiplier, or amplifier. The gain of the amplifier is determined by the ratio of the input and feedback resistors.
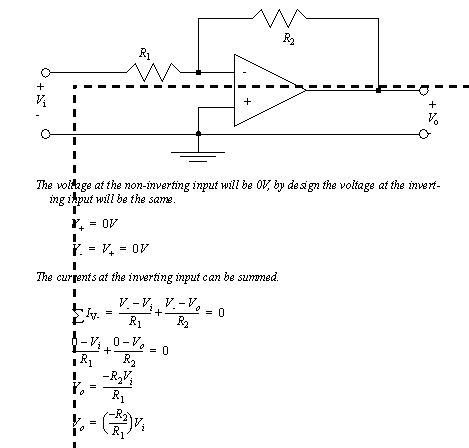
An op-amp circuit that can subtract signals is shown in Figure 7.29 Op-amp example.
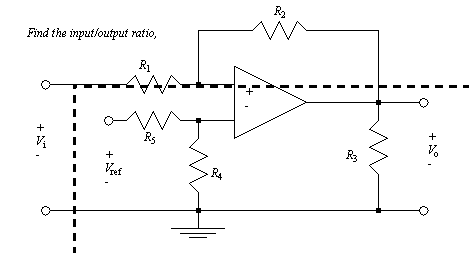
For ideal op-amp problems the node voltage method is normally the best choice. The equations for the circuit in Figure 7.29 Op-amp example and derived in Figure 7.30 Op-amp example (continued). The general approach to this solution is to sum the currents into the inverting and non-inverting input nodes. Notice that the current into the op-amp is assumed to be zero. Both the inverting and non-inverting input voltages are then set to be equal. After that, algebraic manipulation results in a final expression for the op-amp. Notice that if all of the resistor values are the same then the circuit becomes a simple subtractor.
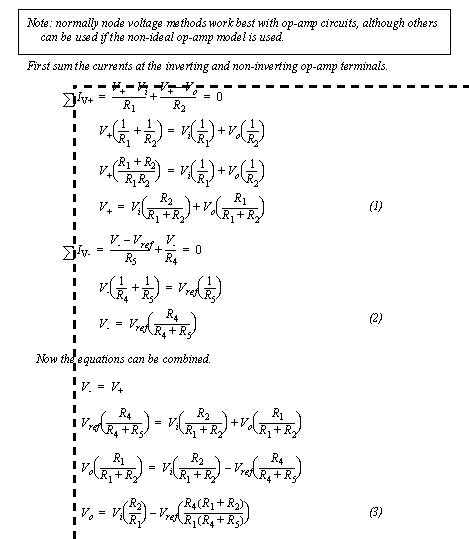
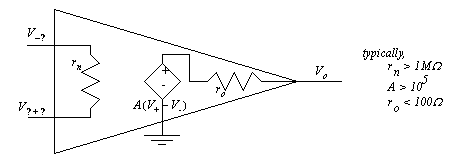
An op-amp (operational amplifier) has an extremely high gain, typically 100,000 times. The gain is multiplied by the difference between the inverting and non-inverting terminals to form an output. A typical op-amp will work for signals from DC up to about 100KHz. When the op-amp is being used for high frequencies or large gains, the model of the op-amp in Figure 7.31 A non-ideal op-amp model should be used. This model includes a large resistance between the inverting and non-inverting inputs. The voltage difference drives a dependent voltage source with a large gain. The output resistance will limit the maximum current that the device can produce, normally less than 100mA.