Engineer On A Disk

8. Forces and Moments Acting on Rigid Bodies

• In real systems we have bodies that have both moments and forces applied. All must total zero for a static case.

• The methods for analyzing these systems requires a good free body diagram. This diagram will be drawn using the forces applied to the system. The reaction forces are put on (as variable in most cases) by evaluating the connections.

8.1 Reactions and Supports

• These symbols are very important to engineers. Their equivalent is the symbols for resistors, transistors, etc in electrical engineering. They are a way to talk about systems in general terms before investing time and resources designing and building the system.

• A subset of these symbols are,

• Other joints include

spring

rod on a smooth surface

rod on a rough surface

pin supports

beams cemented into walls (fixed support)

ball (3D)

ball socket

universal joint

hinge

collar and shaft

8.2 Equilibrium of Forces and Moments

• It is often necessary to solve problems using both sums of moments and forces. Indeed, both of these sums must be equal to zero for the body to be static.

• An example of a problem that has both forces and moments is given below. ([Hibbeler, 1992], prob 5-4, pg.) For the beam suspended by a cable, with a mass at the end, find the forces in the cable, and at point A.

[working model file]

Problem 8.1 Given the force at C and couple at B, find the magnitude and direction of the reaction forces at the supports A and E below.

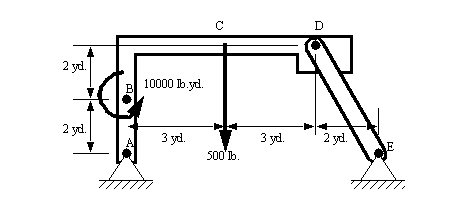
Answer 8.1

• Consider the pictures of both the hoists below, they are much like the examples that follow. The red dampers apply a force that is almost static to slow the lowering of the upper arms.

[picture]

• Lets consider another complex system to solve. In this case free body diagrams are beginning to become essential. ([Hibbeler, 1992], prob 5-44, pg. )

[working model file]

• Now, lets try a problem using vectors, ([Hibbeler, 1992], prob 5-83, pg. )

8.3 Special Cases

• There are a number of standard load cases that we will encounter. One of the simplest is the two force member discussed before.

[picture]

• Three force members can be solved quickly using force triangles, We can also keep in mind that the forces must act concurrently through a common point on the rigid body, or else all three must be parallel.

[picture]

8.3.1 References

8.1 Hibbeler, R.C., Engineering Mechanics: Statics and Dynamics, 6th edition, MacMillan Publishing Co., New York, USA, 1992.

8.4 Statically Indeterminate

• There are a number of problems that cannot be solved with statics methods. In fact, all problems are inexact, but luckily we can solve some using statics.

• These types of problems often occur when the number of forces known is equal to the number of useful governing equations. This is known as completely constrained.

• When there are more equations than unknowns the problem may be over-constrained.

• When there are fewer equations than unknown forces, the problem may be under-constrained.

• The basic types of cases are,

• be cautious with this class of problems. You may actually be able to calculate a solution, but the answer will not be correct realistically, and mathematically speaking.

• Consider problem 2.4-1 from Hibbler