3.8.1 Partial Fractions

 

• The next is a flowchart for partial fraction expansions.

 

 

• The partial fraction expansion for,

 

 

• Consider the example below where the order of the numerator is larger than the denominator.

 

 

• When the order of the denominator terms is greater than 1 it requires an expanded partial fraction form, as shown below.

 

 

• We can solve the previous problem using the algebra technique.

 

 

 

 

3.8.2 Summation and Series

 

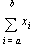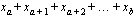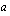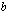• The notation is equivalent to assuming and are integers and 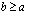. The index variable 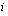is an index often replaced with j, k, m, and n.

 

• Operations on summations:

 

 

 

 

 

 

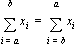
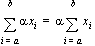
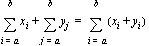
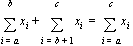
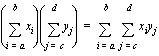
• Some common summations:

 

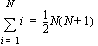
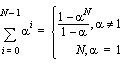for both real and complex .

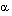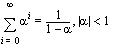for both real and complex . For 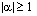, the summation does not converge.

• In Scilab